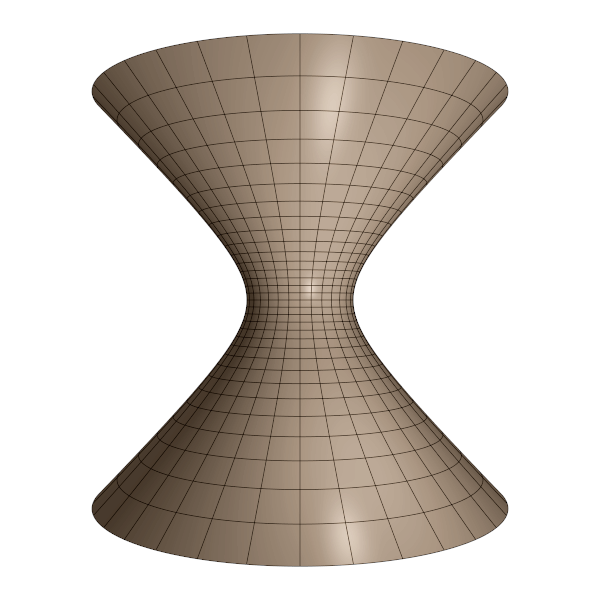
Hyperboloid of one sheet
Object type: Surface
Definition
In $\mathbb{R}^3$, a hyperboloid of one sheet (or one-sheeted hyperboloid) is a set of points $(x,y,z)$ satisfying the equation $$\left(\frac{x}{a}\right)^2 + \left( \frac{y}{b}\right)^2 - \left(\frac{z}{c}\right)^2 = 1$$ for some constants $a, b, c > 0$.
Each intersection with a plane $z = c_0$ yields an ellipse and intersections with $x = 0$ or $y = 0$ yield hyperbolae. Therefore, it is also called an elliptic hyperboloid. If $a = b$, the surface is a hyperboloid of revolution, or a circular hyperboloid, and is obtained by rotating the hyperbola at $x = 0$ or $y = 0$ about the $z$ axis.
Parameterisation
Consider the case of a circular hyperboloid (that is, set $a = b$). This surface is obtained as the hyperbola $$H := \left\{(x,y,z)\in\mathbb{R}^3: \left(\frac{x}{a}\right)^2 - \left(\frac{z}{c} \right)^2 = 1 \wedge x \ge 0 \wedge y = 0\right\}$$ is rotated about the $z$ axis. This hyperbola may be parameterised in two ways. Indeed, $$H = \mathbf{a}(\mathbb{R}) = \mathbf{b}(\mathbb{R})$$ where $$\mathbf{a}: t \mapsto (a \cosh t, 0, c \sinh t)$$ and $$\mathbf{b}: t \mapsto (a\sqrt{1+t^2}, 0, ct).$$ Finally, the general one-sheeted hyperboloid is obtained from the circular one-sheeted hyperboloid by a simple scaling. Therefore, the one-sheeted hyperboloid is the image $\mathbf{r}(\mathbb{R}\times\left[0, 2\pi\right[)$ where $$\mathbf{r}(u,v) = \underline{\mathbf{e}}\begin{pmatrix}a \cosh{u} \cos{v}\\b\cosh{u}\sin{v} \\c\sinh{u}\end{pmatrix},$$ which equals the image $\mathbf{q}(\mathbb{R}\times\left[0,2\pi\right[)$ where $$\mathbf{q}(u,v) = \underline{\mathbf{e}}\begin{pmatrix}a \sqrt{u^2+1} \cos{v}\\b\sqrt{u^2+1}\sin{v} \\cu\end{pmatrix}.$$ The image above shows the parameter curves of $\mathbf{r}$.
Properties (with respect to $\mathbf{r}$)
All properties below are given with respect to the parameterisation $\mathbf{r}$ given above.
Parameter-curve tangent vectors
The parameter-curve tangent vectors are $$\mathbf{r}_u(u,v) = \underline{\mathbf{e}} \begin{pmatrix} a\sinh u\cos v\\b\sinh u\sin v\\c\cosh u\end{pmatrix}, \quad\quad \mathbf{r}_v(u,v) = \underline {\mathbf{e}} \begin{pmatrix}-a\cosh u\sin v\\b\cosh u\cos v\\0\end{pmatrix}.$$
Standard unit normal
The standard unit normal vector field is $$\mathbf{\hat{N}}(u,v) = \frac{1}{\sqrt{b^2 c^2 \cosh^4 u \cos^2 v+a^2 c^2 \cosh^4 u\sin^2 v+a^2 b^2 \sinh^2 u\cosh^2 u}} \underline{\mathbf{e}}\begin{pmatrix} -bc\cosh^2 u\cos v\\-ac\cosh^2 u\sin v\\ab\sinh u\cosh u\end{pmatrix}$$ which, in the case of a circular hyperboloid, reduces to $$\mathbf{\hat{N}}(u,v) = \frac{1}{\sqrt{c^2\cosh^2 u+a^2\sinh^2 u}} \underline{\mathbf{e}}\begin{pmatrix}-c\cosh u\cos v\\-c\cosh u\sin v\\a\sinh u\end{pmatrix}.$$
Area element
The area element is $$dA = \sqrt{b^2 c^2 \cosh^4 u\cos^2 v+a^2 c^2 \cosh^4 u\sin^2 v+a^2 b^2 \sinh^2 u\cosh^2 u}~dudv$$ which, in the case of a circular hyperboloid, reduces to $$dA = a\cosh{u}\sqrt{c^2\cosh^2 u+a^2\sinh^2 u}~dudv.$$
First fundamental form
The first fundamental form is $$\mathcal{F}(u,v) = \begin{pmatrix}a^2 \sinh^2 u\cos^2 v+b^2 \sinh^2 u\sin^2 v+c^2\cosh^2 u&&(b^2-a^2)\sinh u\cosh u\sin v\cos v\\(b^2-a^2)\sinh u\cosh u\sin v\cos v&&a^2 \cosh^2 u\sin^2 v + b^2\cosh^2 u\cos^2 v\end{pmatrix}$$ which, in the case of a circular hyperboloid, reduces to $$\mathcal{F}(u,v) = \begin{pmatrix}a^2\sinh^2 u+c^2\cosh^2 u&&0\\0&&a^2\cosh^2 u\end{pmatrix}.$$
Second fundamental form
The second fundamental form is $$\mathcal{M}(u,v) = \frac{abc\cosh u}{\sqrt{b^2 c^2 \cosh^4 u\cos^2 v+a^2 c^2 \cosh^4 u\sin^2 v+a^2 b^2 \sinh^2 u\cosh^2 u}}\begin{pmatrix}-1&&0\\0&&\cosh^2 u\end{pmatrix}$$ which, in the case of a circular hyperboloid reduces to $$\mathcal{M}(u,v) = \frac{ac}{\sqrt{c^2\cosh^2 u+a^2\sinh^2 u}}\begin {pmatrix}-1&&0\\0&&\cosh^2 u\end{pmatrix}.$$
Christoffel symbols
In the case of a circular hyperboloid ($a=b$), the Christoffel symbols are $$\Gamma^1_{\alpha\beta} = \frac{\tanh u}{a^2\tanh^2 u+c^2}\begin{pmatrix}a^2+c^2&&0\\0&&-a^2\end{pmatrix}, \quad\quad\Gamma^2_{\alpha\beta} = \tanh u\begin{pmatrix}0&&1\\1&&0\end{pmatrix}.$$
Curvatures
In the case of a circular hyperboloid ($a=b$), the principal curvatures are $$\kappa_1 = \frac{-ac} {\left(a^2 \sinh^2 u+c^2 \cosh^2 u\right)^{3/2}}, \quad\quad \kappa_2 = \frac{c}{a\sqrt{a^2 \sinh^2 u+c^2 \cosh^2 u}}$$ in the directions $(1,0)$ and $(0,1)$, respectively, and so the Gaussian and mean curvatures are $$K = \frac{-c^2}{\left(a^2\sinh^2 u+c^2\cosh^2 u\right)^2}, \quad\quad H = \frac{c^3\cosh^2u+a^2 c\sinh^2 u−a^2 c} {a\left(a^2\sinh^2 u+c^2\cosh^2 u\right)^{3/2}}.$$
Properties (with respect to $\mathbf{q}$)
All properties below are given with respect to the parameterisation $\mathbf{q}$ given above.
Parameter-curve tangent vectors
The parameter-curve tangent vectors are $$\mathbf{q}_u(u,v) = \underline{\mathbf{e}}\begin{pmatrix} au\left(u^2+1\right)^{-1/2}\cos{v}\\bu\left(u^2+1\right)^{-1/2}\sin{v}\\c\end{pmatrix}, \quad\quad \mathbf{q}_v(u,v) = \underline{\mathbf{e}}\begin{pmatrix}-a\sqrt{u^2+1}\sin{v}\\b\sqrt{u^2+1}\cos{v}\\ 0\end{pmatrix}.$$
Standard unit normal
The standard unit normal vector field is $$\mathbf{\hat{N}}(u,v) = \frac{1}{\sqrt{b^2 c^2(u^2+1)\cos^2 v + a^2 c^2(u^2+1)\sin^2 v + a^2 b^2 u^2}}\underline{\mathbf{e}}\begin{pmatrix}-bc\sqrt{u^2+1}\cos{v}\\-ac \sqrt{u^2+1}\sin{v}\\abu\end{pmatrix}.$$ In the case of a circular hyperboloid, this reduces to $$\mathbf {\hat{N}} = \frac{1}{\sqrt{(a^2+c^2)u^2+c^2}}\underline{\mathbf{e}}\begin{pmatrix}-c\sqrt{u^2+1}\cos{v}\\ -c\sqrt{u^2+1}\sin{v}\\au\end{pmatrix}.$$
Area element
The area element is $$dA = \sqrt{b^2 c^2(u^2+1)\cos^2 v + a^2 c^2(u^2+1)\sin^2 v + a^2 b^2 u^2}~dudv.$$ In the particular case of a circular hyperboloid, this reduces to $$dA = a\sqrt{(a^2+c^2)u^2+c^2}~dudv.$$
First fundamental form
The first fundamental form of the one-sheeted hyperboloid is $$\mathcal{F}(u,v) = \begin{pmatrix}a^2 u^2 (u^2 + 1)^{-1} \cos^2{v} + b^2 u^2 (u^2 + 1)^{-1} \sin^2 {v} + c^2&&(b^2 - a^2)u\sin{v}\cos{v}\\(b^2 - a^2)u \sin{v}\cos{v}&&a^2(u^2+1)\sin^2 v + b^2(u^2 + 1)\cos^2 v\end{pmatrix}$$ which, in the case of a circular hyperboloid, reduces to $$\mathcal {F}(u,v) = \begin{pmatrix}a^2 u^2 (u^2 +1)^{-1} + c^2&&0\\0&&a^2(u^2+1) \end{pmatrix}.$$
Second fundamental form
The second fundamental form is $$\mathcal{M}(u,v) = \frac{abc}{\sqrt{b^2 c^2(u^2+1)\cos^2 v + a^2 c^2(u^2+1) \sin^2 v + a^2 b^2 u^2}}\begin{pmatrix}-(u^2 + 1)^{-1}&&0\\0&&u^2+1\end{pmatrix}.$$ In the case of a circular hyperboloid, this reduces to $$\mathcal{M}(u,v) = \frac{ac}{\sqrt{(a^2+c^2)u^2+c^2}} \begin{pmatrix}-(u^2 + 1)^{-1}&&0\\0&&u^2+1\end{pmatrix}.$$
Christoffel symbols
In the case of a circular hyperboloid ($a = b$), the Christoffel symbols are $$\Gamma^1_{\alpha\beta} = \frac{a^2 u}{(a^2+c^2)u^2+c^2}\begin{pmatrix}\frac{1}{u^2+1}&&0\\0&&-(u^2+1)\end{pmatrix}, \quad\quad \Gamma^2 _{\alpha\beta} = \frac{u}{u^2+1}\begin{pmatrix}0&&1\\1&&0\end{pmatrix}.$$
Curvatures
In the case of a circular hyperboloid ($a = b$), the principal curvatures are $$\kappa_1 = \frac{-ac}{\left( (a^2+c^2)u^2+c^2\right)^{3/2}}, \quad\quad\kappa_2 = \frac{c} {a\sqrt{(a^2+c^2)u^2+c^2}}$$ with principal directions $(1, 0)$ and $(0, 1)$, respectively. Hence, the Gaussian and mean curvatures are $$K = \frac{-c^2} {\left((a^2+c^2)u^2+c^2\right)^2}, \quad\quad H = \frac{(a^2+c^2)cu^2+c^3-a^2c}{a\left((a^2+c^2)u^2 +c^2\right)^{3/2}}.$$